Carbon 14 Dating Calculator
Carbon 14 Dating Calculator and Problems dating Arrival of the Aboriginal Peoples in Australia
Carbon 14 Dating Calculatorpresented by Earth Science Australia through the kind permission of the author Mark GregoryTo find the percent of Carbon 14 remaining after a given number of years, type in the number of years and click on Calculate.
To find the years that have elapsed from how much Carbon 14 remains, type in the C 14 percent and click on Calculate.
|
More about Carbon Dating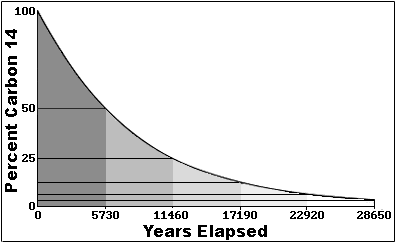 In the 1940's Dr. Willard F. Libby invented carbon dating for which he received the Nobel Prize in chemistry in 1960. Carbon dating has given archeologists a more accurate method by which they can determine the age of ancient artifacts. The halflife of carbon 14 is 5730 ± 30 years, and the method of dating lies in trying to determine how much carbon 14 (the radioactive isotope of carbon) is present in the artifact and comparing it to levels currently present in the atmosphere. Above is a graph that illustrates the relationship between how much Carbon 14 is left in a sample and how old it is.
|
The problem of Dating the Arrival of the Aboriginal Peoples of Australia
Radio Carbon Dating of +40000 year old materials – errors involved
Early atmospheric nuclear testing upset the global uniformity carbon 14-12 ratios. For radio carbon testing by future archaeologists, “Year Zero” ( January 1st 1950) is considered the last date sufficiently unaffected to be useful for dating.
There is no exact date beyond which carbon 14 decay is/is not useful. But, given that the half life of carbon 14 is 5730 years, then there really isn't much carbon 14 left in a sample that is 40,000 years old.
The decay constant is λ=ln2/t1/2
so the fraction of carbon 14 left would be exp[−λt], which, for t=40,000 years,
would be 0.79%.
Of course, these small traces probably could be found with modern techniques, with some uncertainty, but then you have to factor in systematic uncertainties - for example associated with present-day contamination (the air contains carbon 14 !).
Any small uncertainty in the measurements, in the amount of contamination (or any other source of small error such as fluctuations in the naturally occurring 14 to 12 C ratio) could easily be magnified into a huge age error in an old sample with a very small amount of carbon 14 present.
For example, let's say you can measure the 14/12 C ratio to be f±δf (in a system of units where the original ratio was expected to be 1).
Crudely speaking, what you do next is to extrapolate a decay curve back in time to see how long ago the sample would have had f=1.
Thus: f=exp[−λτ] lnf=−λτ δff=|−λδτ| δτ=t1/2ln2δff
So say your ability to measure f was limited to ±0.02 because of potential contamination or other complications,
then If f=0.5 (i.e something that is just 5730 years old), then your uncertainty would be a perhaps tolerable ±330 years.
However, if f=0.0079 (for 40,000 years old), then the uncertainty would be a less-than-useful ±20,800 years.
In fact, the latter example is worse (more asymmetric) than that, because formula (1) is not valid when δf>f
In reality, the uncertainty is consistent with there being anywhere from no carbon 14 at all (and so an infinite age) to f∼0.028, which would imply τ∼30000 years old.

